Simulation study of groundwater flow and solute transport processes in karst underground rivers based on GMS
-
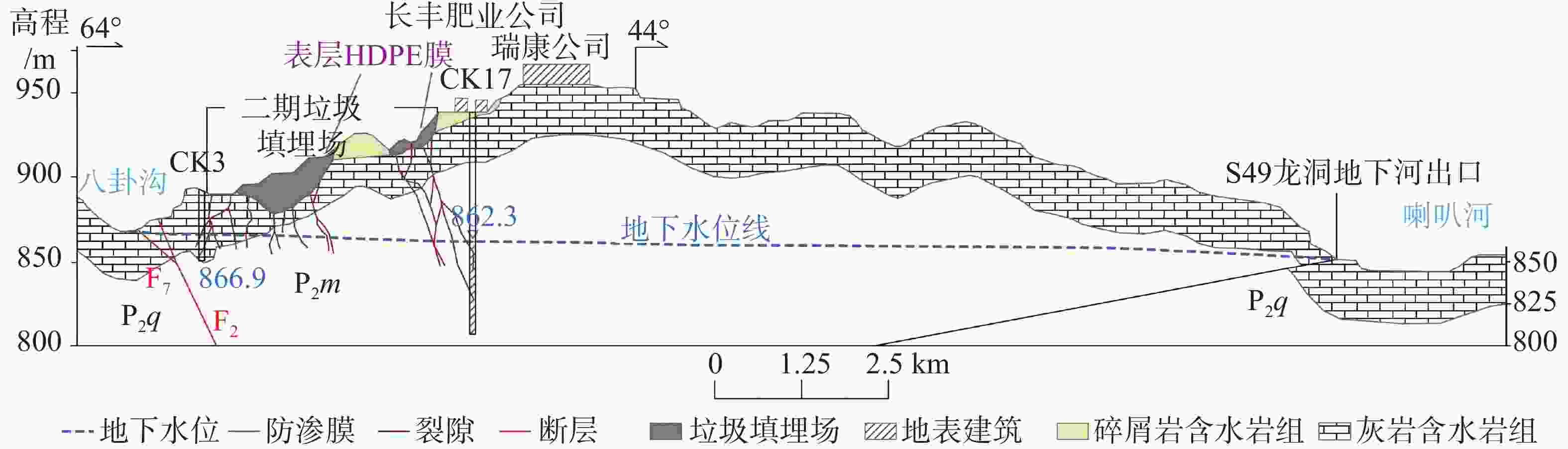
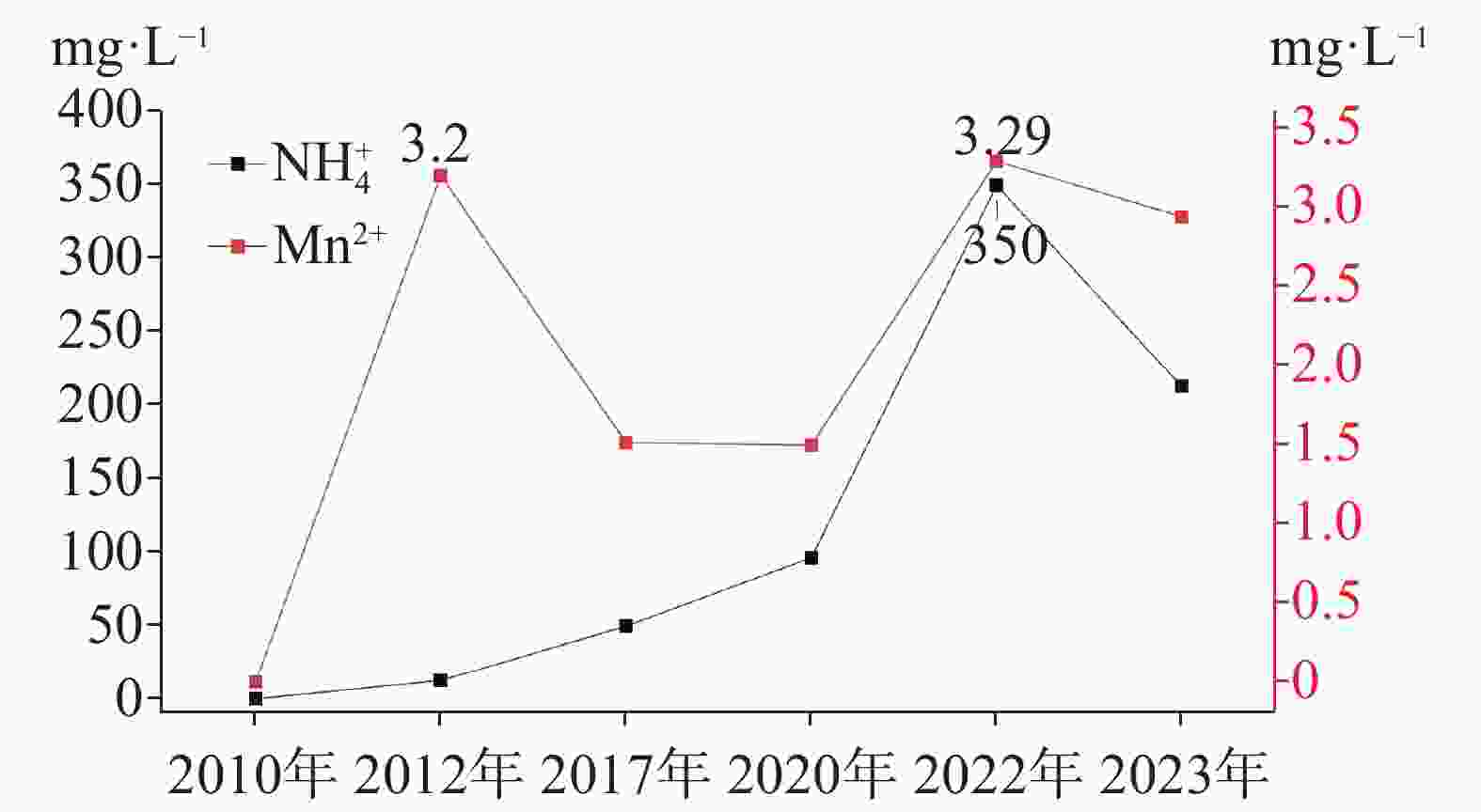
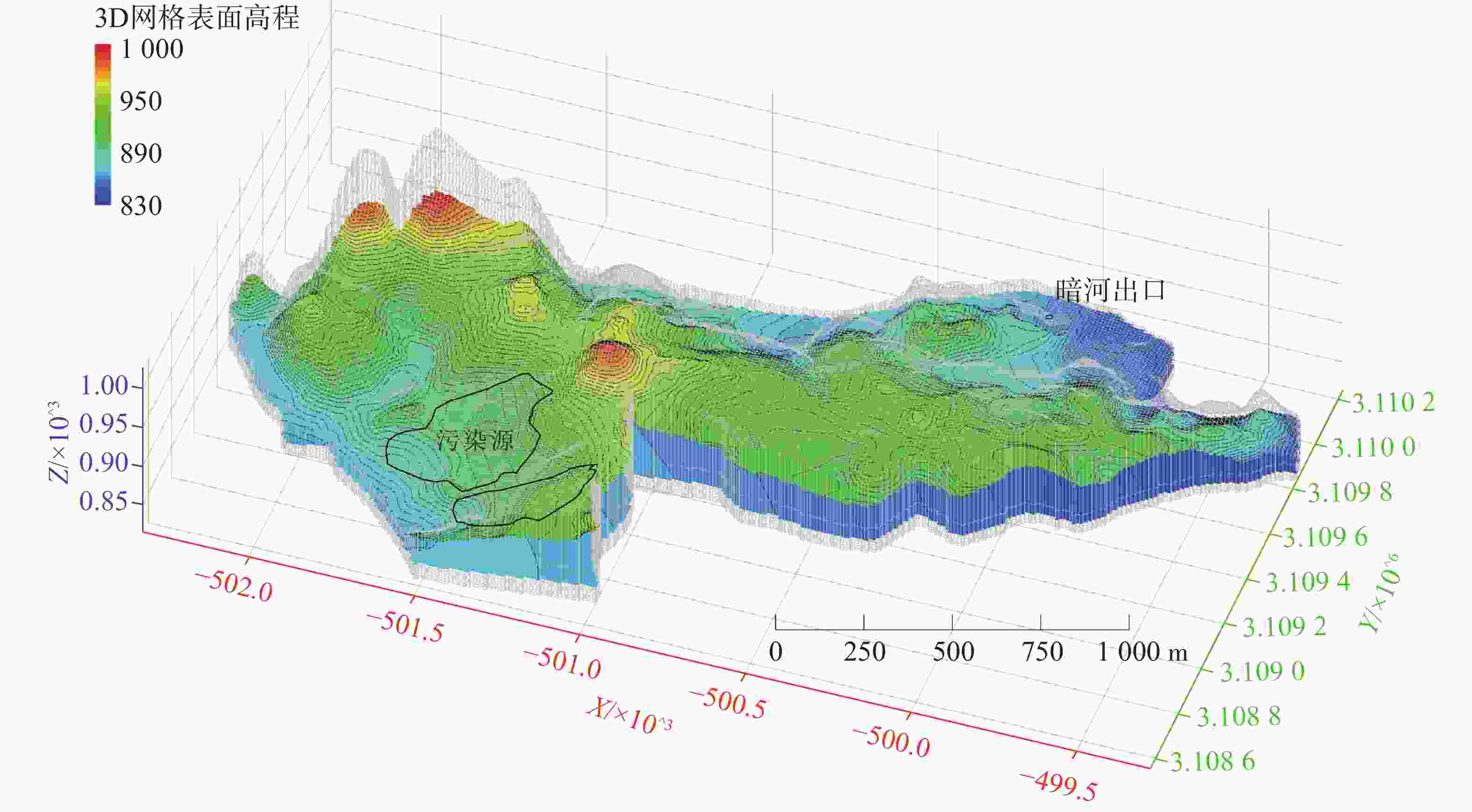
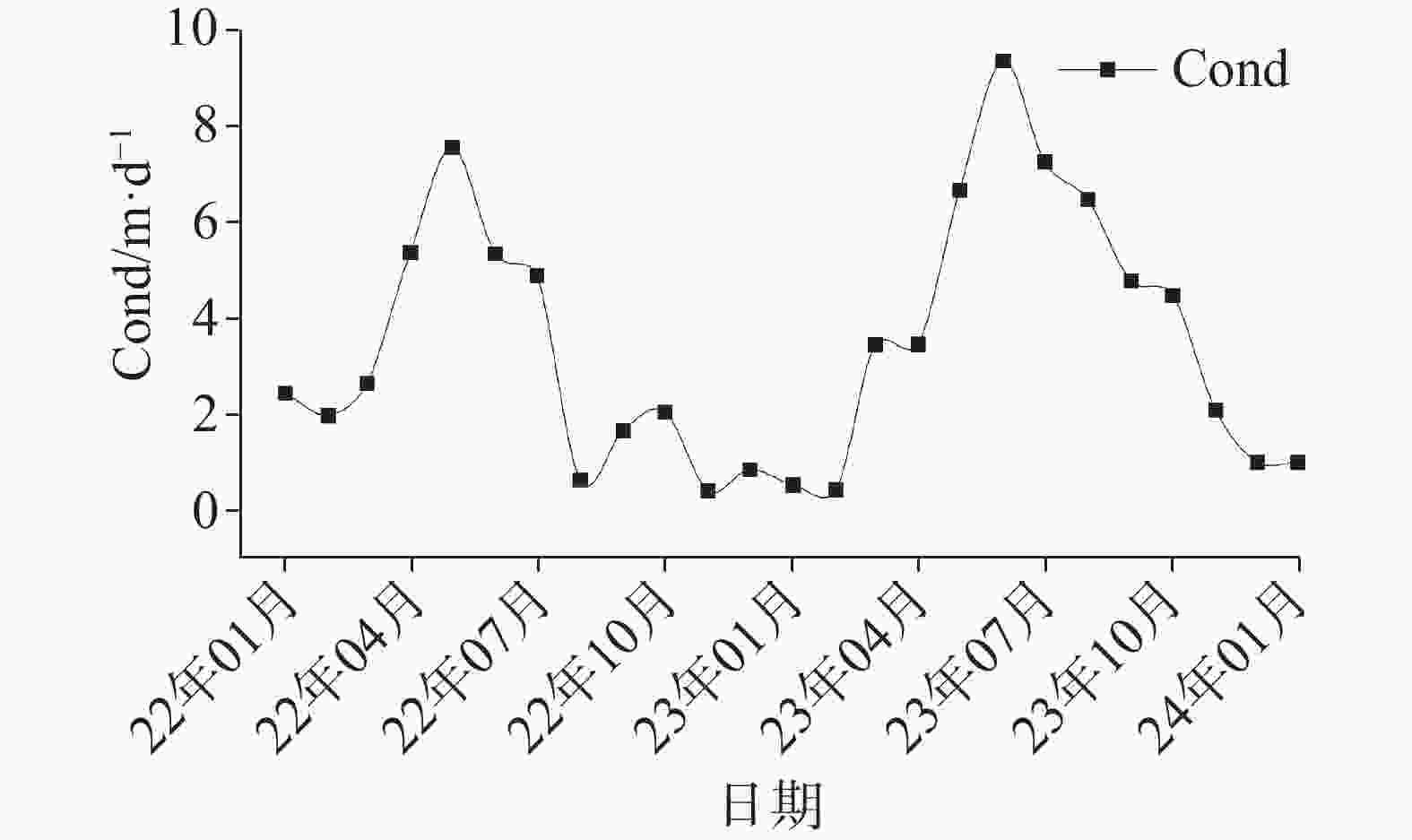
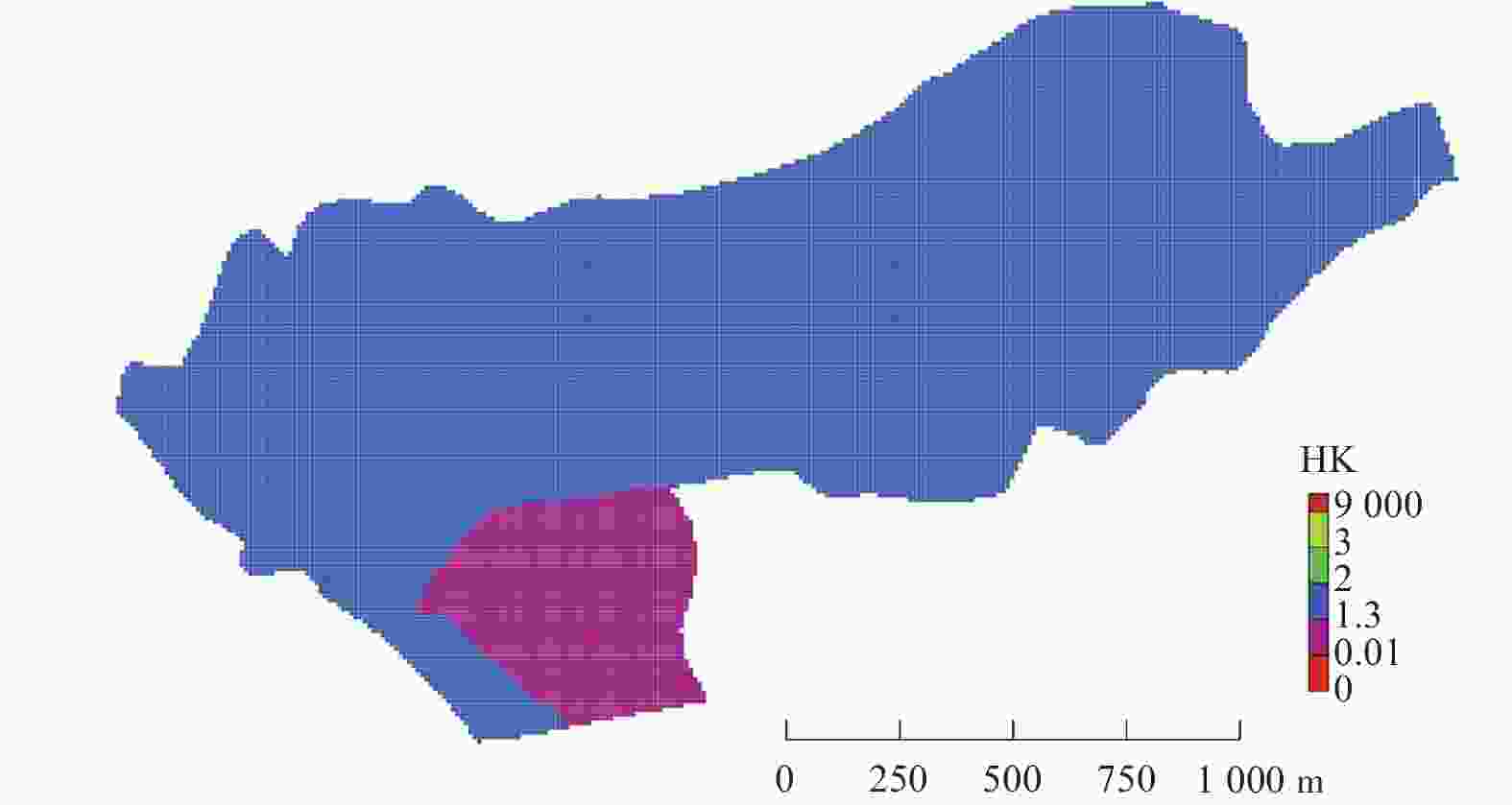
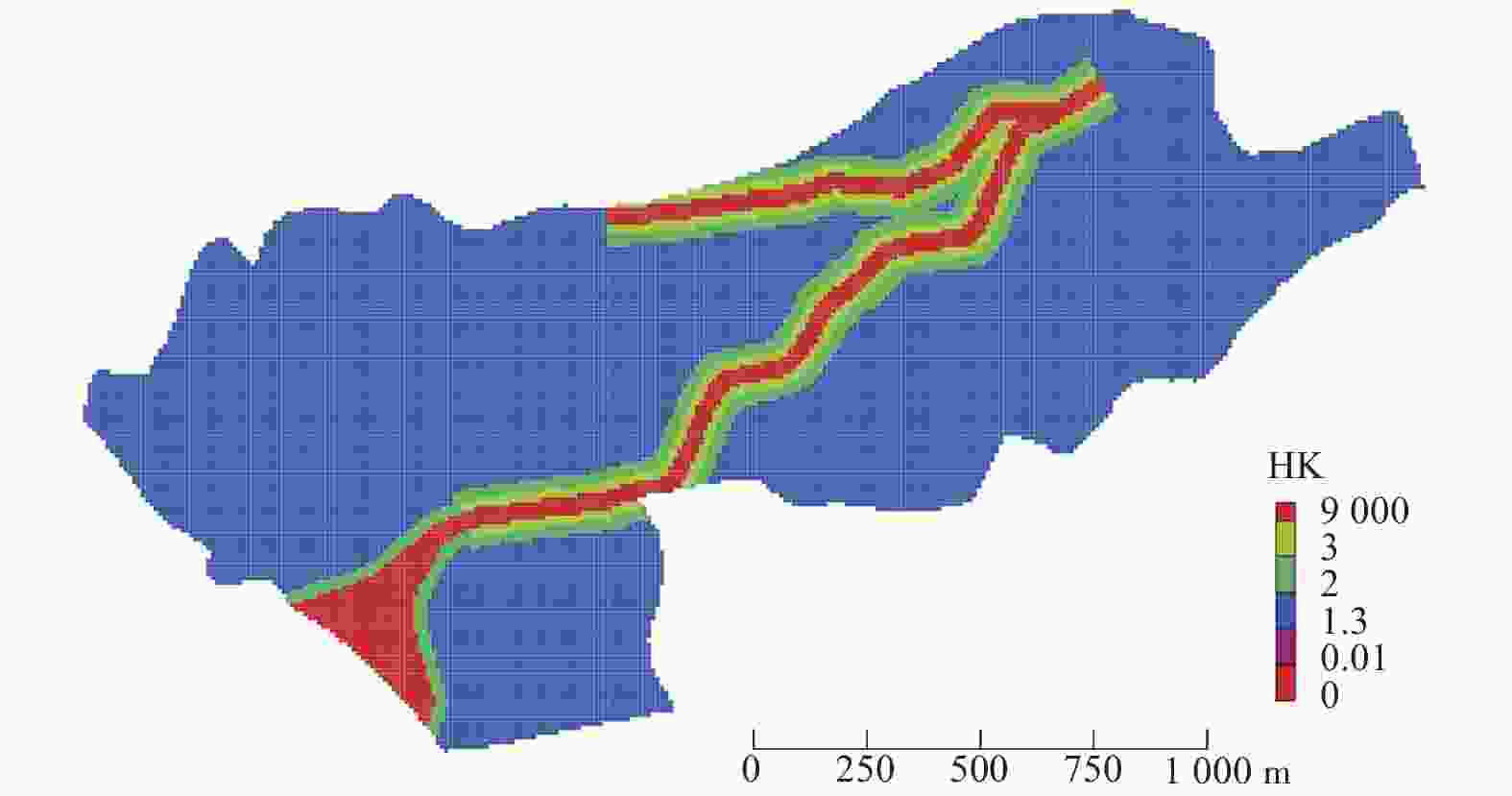
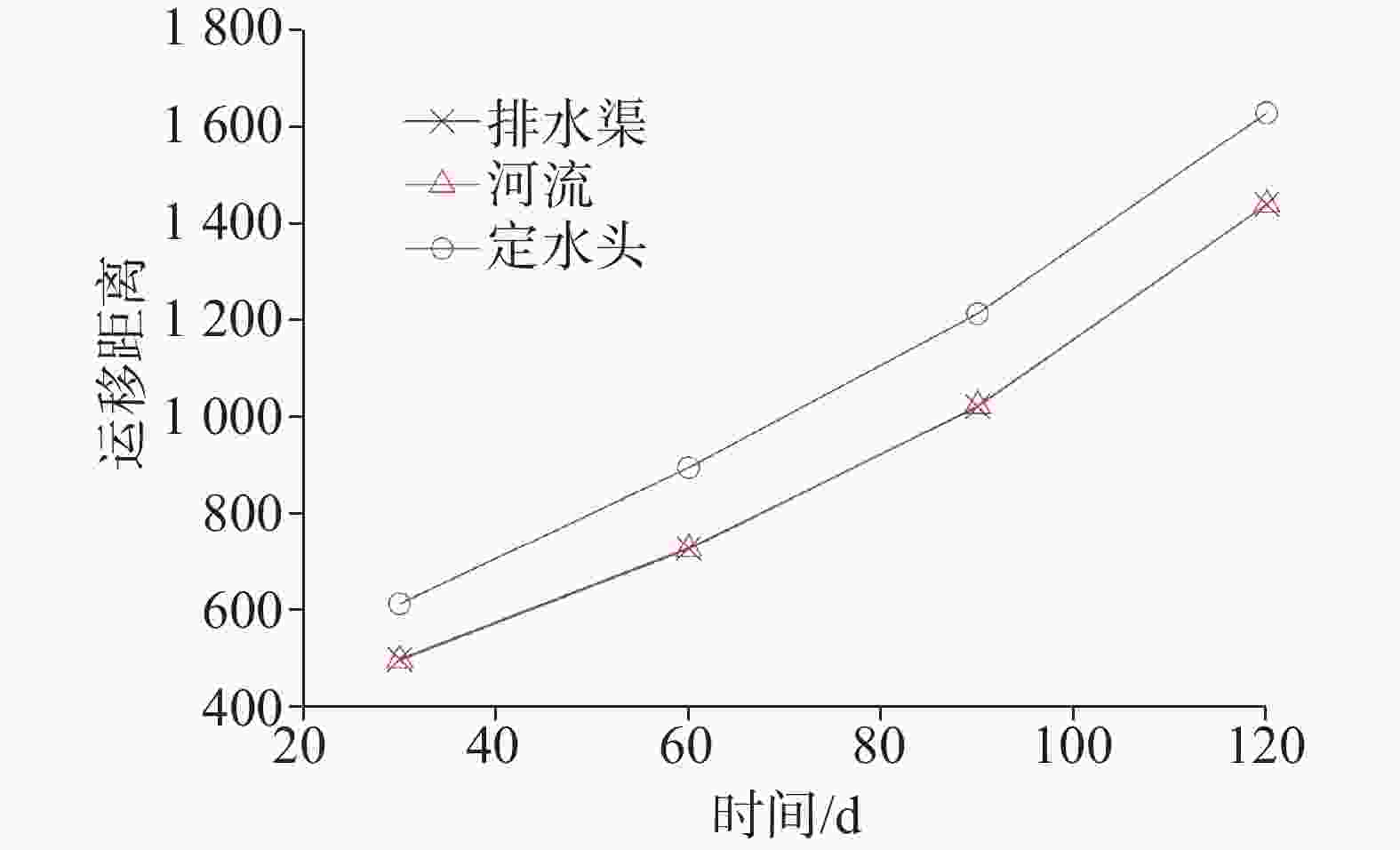
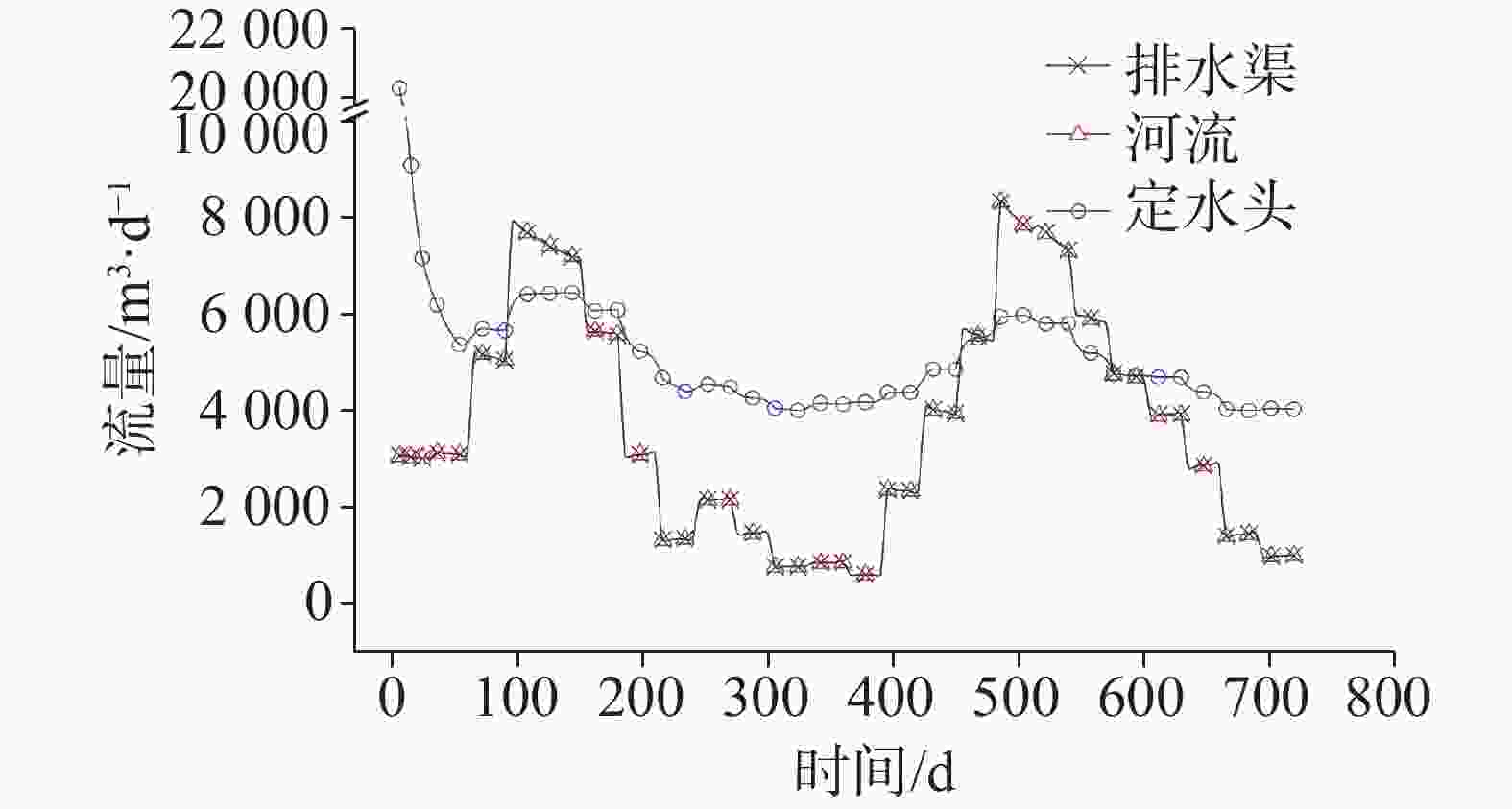
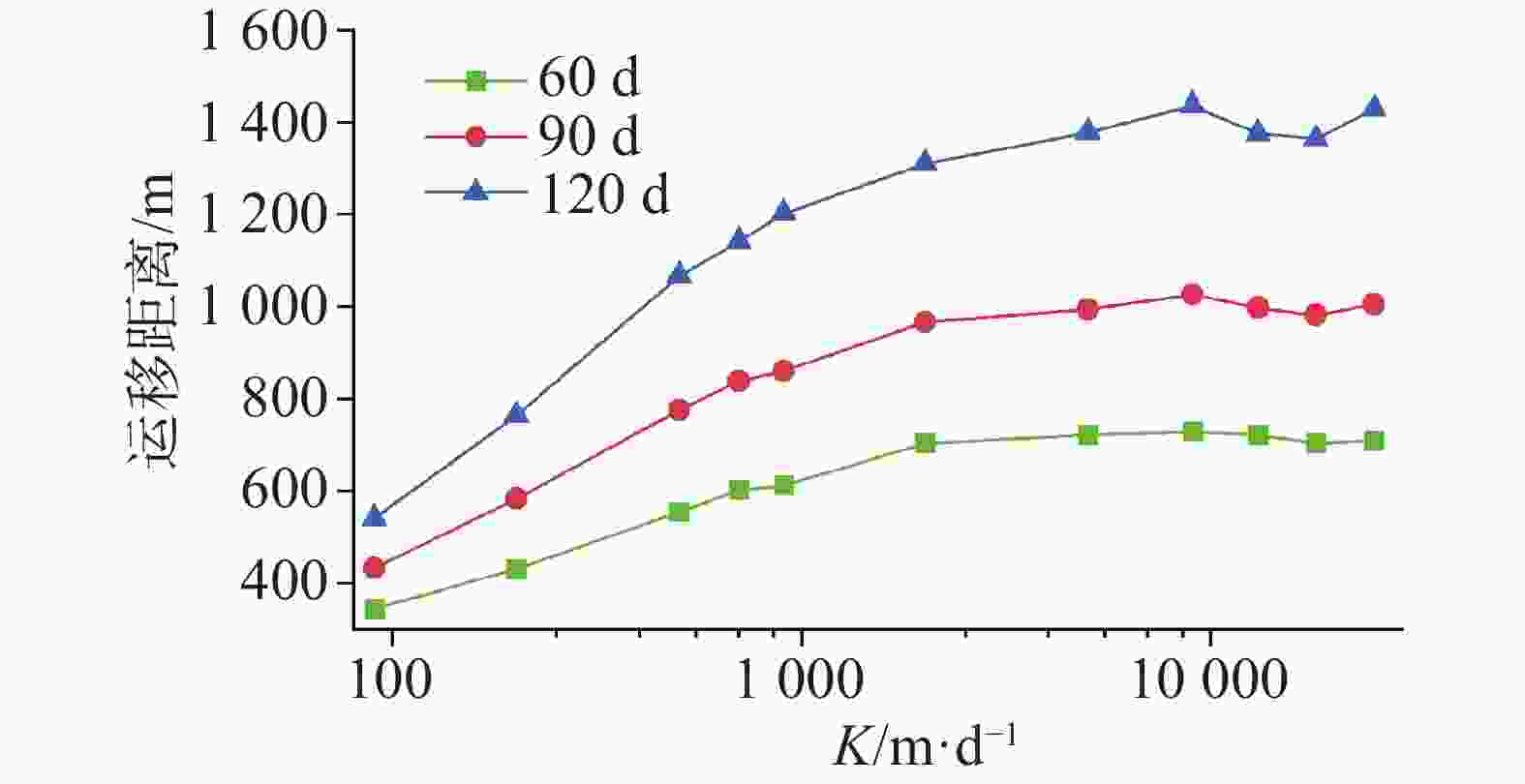
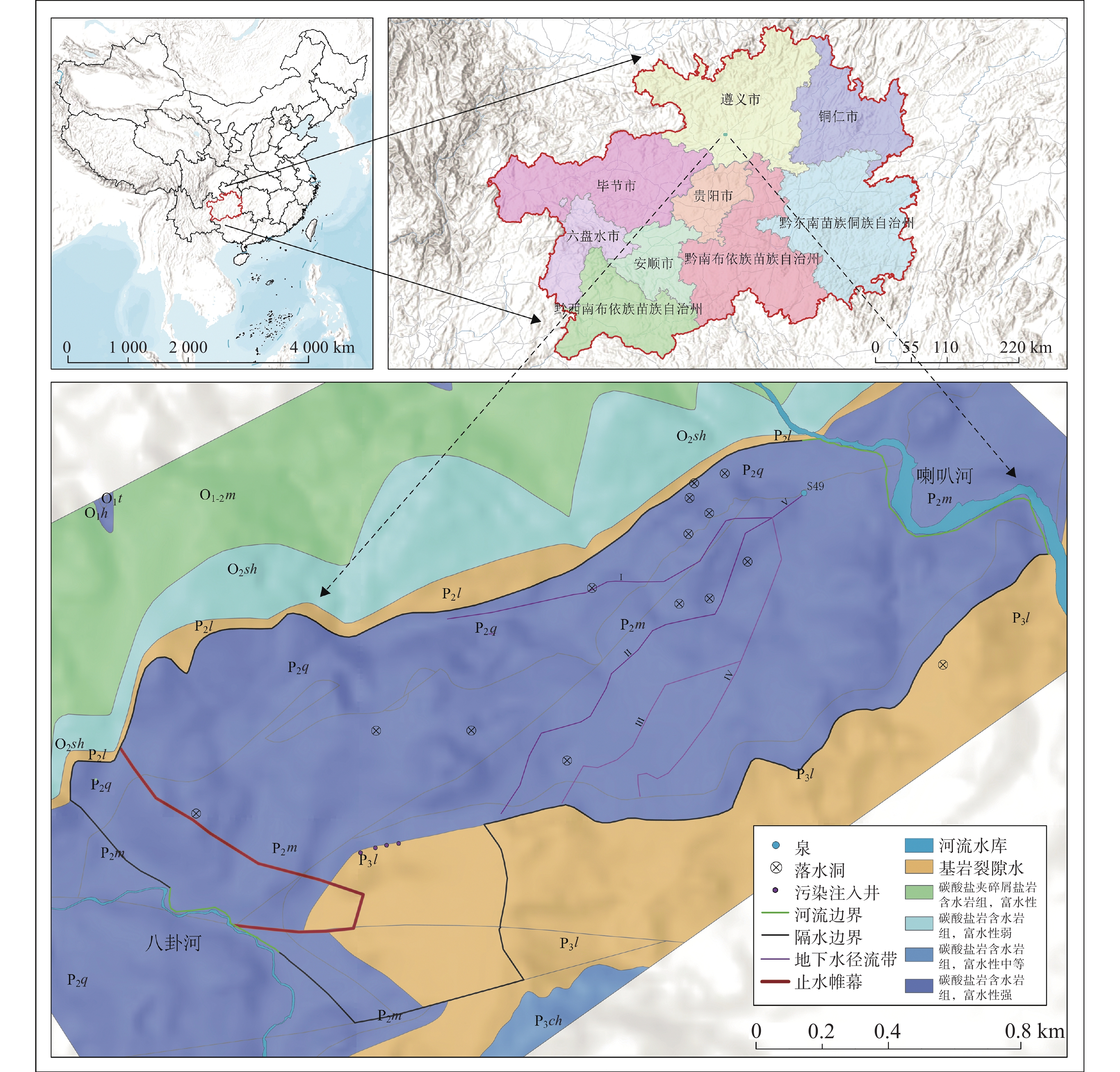
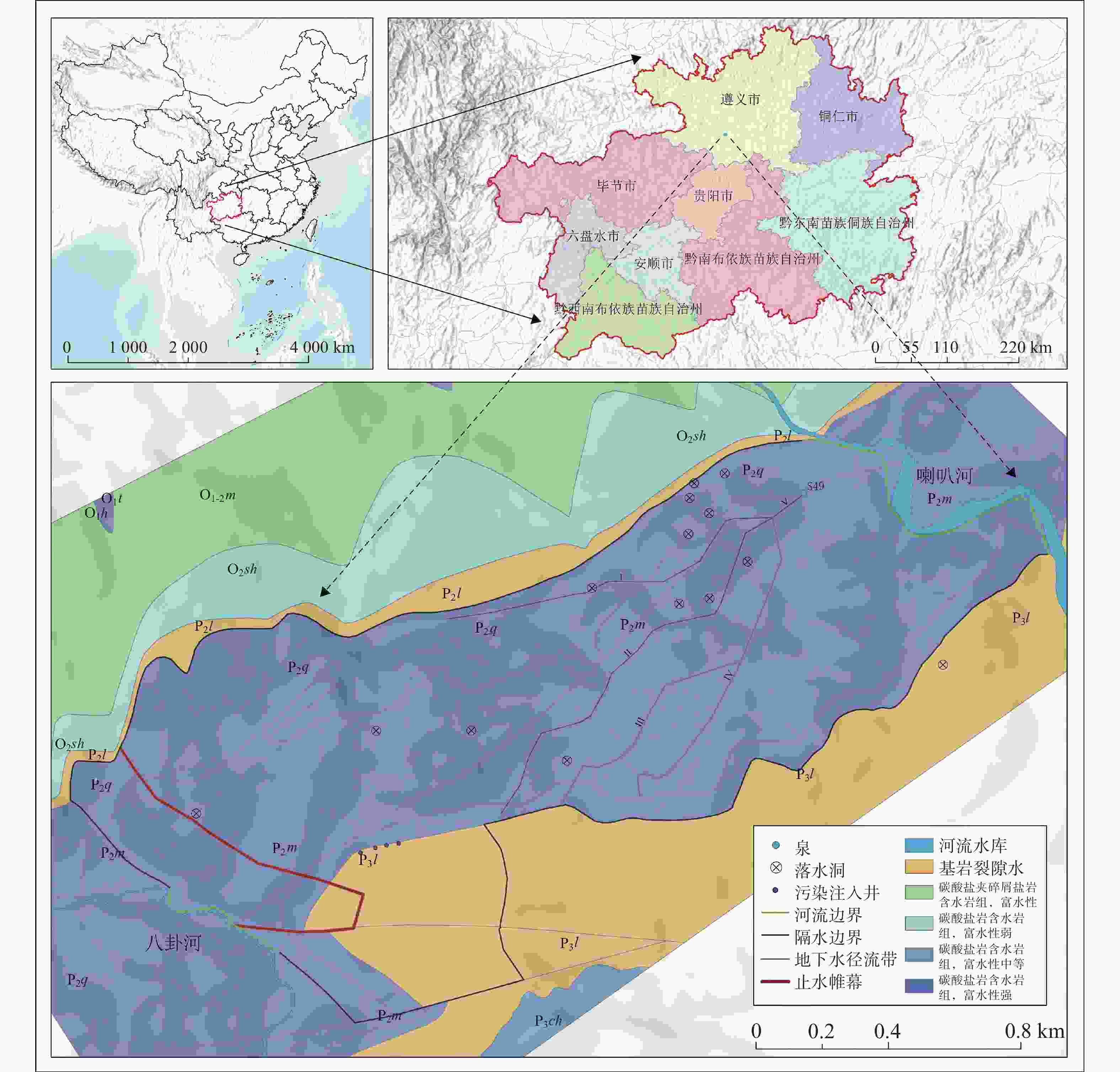
摘要: 文章针对遵义市龙洞地下河系统，采用地下水模拟软件GMS（Groundwater Modeling System）构建数值模型。通过构建大渗透系数K概化岩溶地下河的水流特性，旨在通过较少的参数达成较高的模拟效率；利用2022—2023年地下河出口流量数据进行模型识别和验证，确保模拟结果的可靠性；之后通过示踪试验比较模拟与观测数据，揭示当前溶质运移模拟中存在的时间和空间尺度误差，尤其用大渗透系数达西流表征岩溶地下河特性的局限性。结果表明GMS在模拟岩溶地下水流动方面表现出较好的一致性，但在溶质运移模拟方面的精度有待提高，特别是在渗透系数大(
9000 m·d−1)的情况下，预测结果与实际观测存在一定偏差。通过调整模型渗透系数和设置缓冲带，改善模拟精度，并阐明其对溶质运移范围和速度的显著影响，表明参数优化是提高模型预测准确度的关键。提出针对岩溶地下水模拟的改进措施，包括优化模型的参数设置、引入更复杂的水动力学模型（如EPM、DC和CDC模型）以及提高模型在非达西流动条件下的应用能力。未来应继续探索模型参数的最优化，并通过更多实地验证来提高模型的预测能力和适用性，以期为岩溶区水资源管理和保护提供科学的决策支持。Abstract:As the demand for the development and protection of groundwater resources in karst regions increases, accurately simulating the flow and solute transport characteristics of these waters becomes crucial. This study focuses on the Longdong underground river system in Zunyi City to construct a numercial model, utilizing the Groundwater Modeling System (GMS). By conceptualizing the water flow characteristics of the karst underground river with a high hydraulic conductivity coefficient (K), this study is aimed to achieve high simulation efficiency with fewer parameters. This approach is particularly advantageous in karst areas, where traditional modeling techniques may struggle to capture the complex interactions between water flow and geological features. Given the unique geological structures, karst conduits, and fractures present in these regions, a sophisticated model is necessary for proper conceptualization. The model considers the distinct heterogeneity and anisotropy of karst aquifers, with a particular focus on the complex flow patterns characteristic of conduit-dominated flow. Karst aquifers are known for their irregular and often unpredictable flow paths, which can significantly influence the movement of both water and solutes. This study underscores the importance of understanding these flow patterns, as they are critical for effective water resource management and pollution control in karst environments. To ensure the reliability of the simulation results, model identification and validation were conducted with the use of discharge data from the underground river's outlet from 2022 to 2023. This validation process is essential, as it not only confirms the model's accuracy but also enhances the credibility of its predictive capabilities for future scenarios. By utilizing measured data, the study improves the model's reliability, making it a valuable tool for researchers and pollution analysts. Subsequently, tracer tests were conducted to compare simulated and observed data, revealing temporal and spatial scale errors present in current solute transport simulations, particularly highlighting the limitations of using high hydraulic conductivity Darcy flow to characterize karst underground rivers. Tracer tests are vital in hydrological studies, as they provide insights into the movements of solutes within aquifers, enabling researchers to effectively assess of their models. The discrepancies observed in this study highlight the challenges faced in accurately modeling solute transport in environments with high hydraulic conductivity, where traditional assumptions may no longer hold true. This underscores the need for continuous refinement of modeling techniques to better align with the dynamic nature of karst systems. The findings indicate that GMS demonstrates good consistency in simulating karst groundwater flow; however, there is a need to enhance the accuracy of solute transport simulations, especially under high hydraulic conductivity ( 9000 m/d), where deviations between predicted results and actual observations were noted. These discrepancies emphasize the challenges of accurately modeling solute transport under high hydraulic conductivity conditions, suggesting that reliance on high hydraulic conductivity values may lead to oversimplifications that inadequately represent the complexities of solute movement in karst systems. By adjusting the hydraulic conductivity within the model and implementing buffer zones, simulation accuracy was improved, highlighting the significant impact on the range and velocity of solute transport. This indicates that parameter optimization is key to enhancing the predictive accuracy of the model. The introduction of buffer zones also underscores their potential to mitigate scale-dependent errors, providing a novel approach for managing uncertainty in karst system modeling. This innovative method not only enhances the reliability of the model but also serves as a transitional area that helps to smooth discrepancies between modeled and observed data, thereby facilitating model convergence.Finally, this study proposes several improvements for karst groundwater simulations, including optimizing model parameter settings, incorporating more complex hydrodynamic models (such as EPM, DC, and CDC models), and increasing the model's applicability under non-Darcy flow conditions. Furthermore, the study advocates the integration of other models to better represent the interactions between karst conduits and the surrounding matrix, which could lead to more accurate predictions of both flow and solute transport in complex karst terrains. Future research should prioritize the continued optimization of model parameters and enhance the model's predictive accuracy and applicability through extensive field validation. This will provide robust scientific support for decision-making in the management and conservation of water resources in karst regions. In summary, the development of more refined models to improve the resolution of both macroscopic conduit networks and microscopic fracture systems is essential for advancing karst hydrogeological research. -
Key words:
- GMS /
- groundwater /
- numerical simulation /
- karst conduit
-
图 1 遵义市龙洞地下河岩溶流域交通位置与水文地质图
Figure 1. Traffic location and hydrogeological map of the karst basin of the Longdong underground river in Zunyi City
图 2 龙洞地下河系统污染成因模式示意图
Figure 2. Diagram of the pollution genesis model for the Longdong underground river system
图 6 2022—2023降雨蒸发分布图
Figure 6. Distribution of precipitation and evaporation from 2022 to 2023
图 10 龙洞地下河系统等水位线及流场图
Figure 10. Contour map of water levels and flow field of the Longdong underground river system
图 14 龙洞地下河出口示踪曲线对比结果
Figure 14. Comparison of the tracer curves at the outlet of Longdong underground river
图 15 不同出口概化的污染物运移距离对比图
Figure 15. Comparison of pollutant migration distances for different outlet generalizations
图 17 不同渗透系数下污染物运移情况示意图
Figure 17. Schematic diagram of pollutant transport under different permeability coefficients
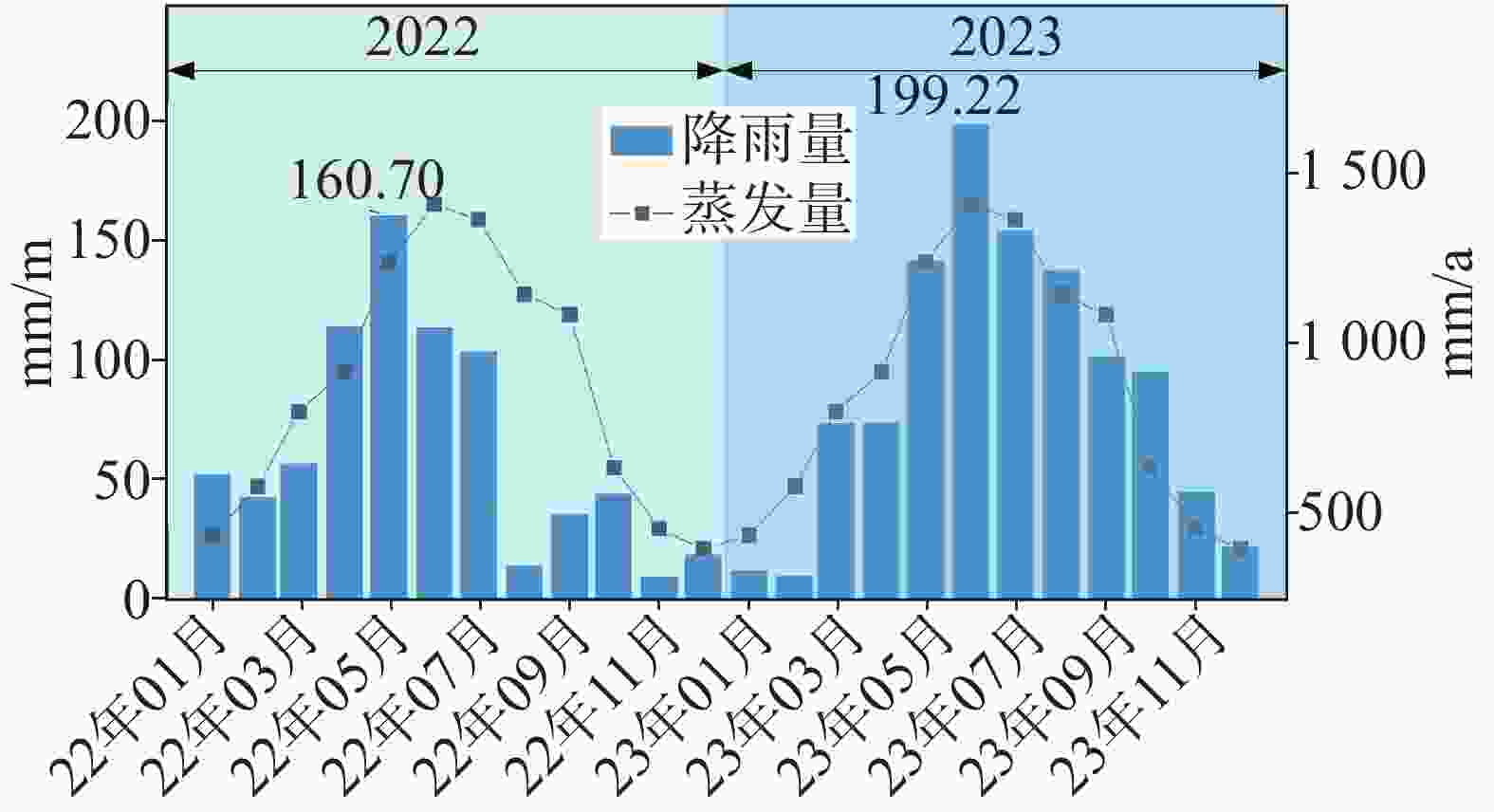
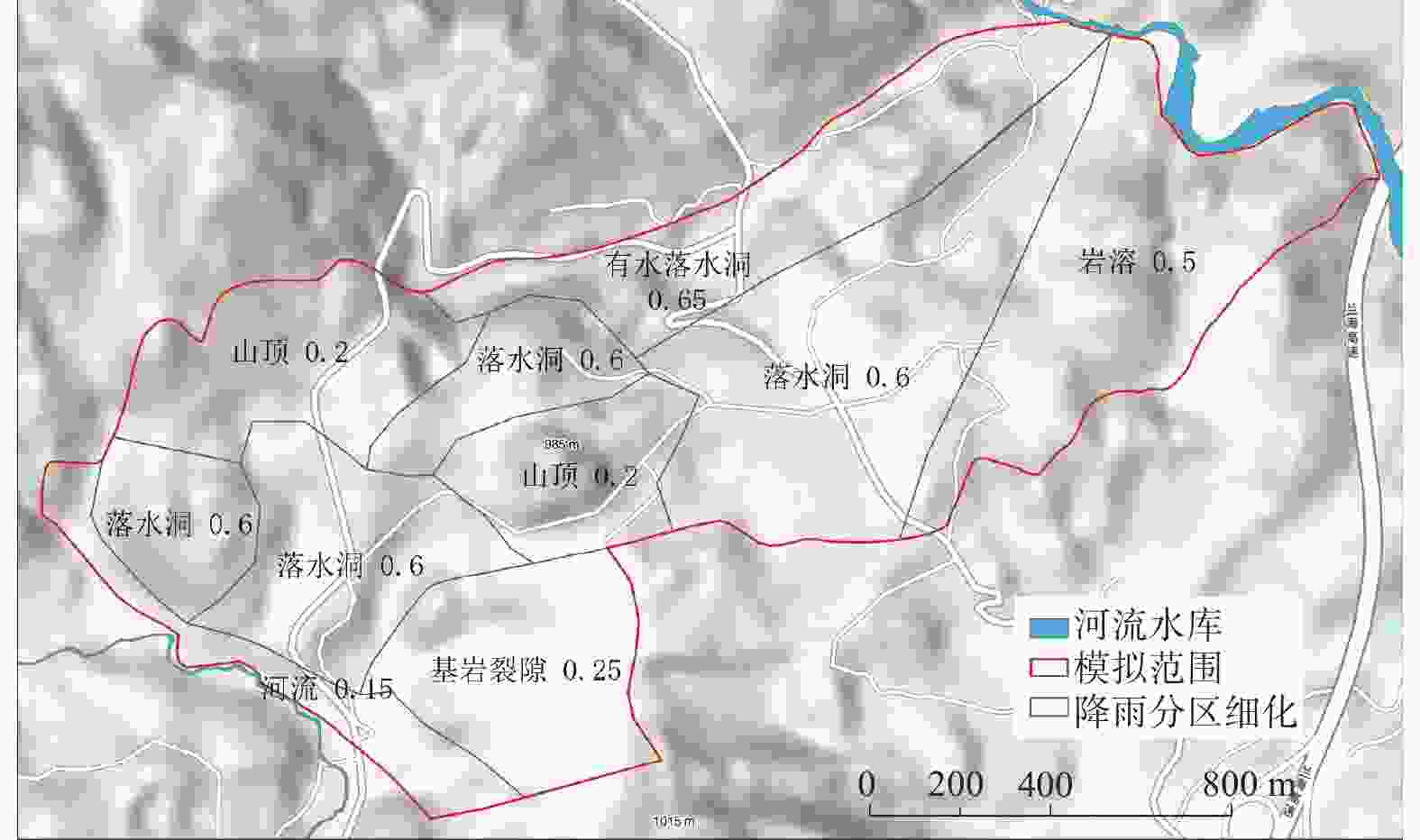
表 1 降雨入渗系数分区表
Table 1. Zoning of rainfall infiltration coefficient
分区依据 降雨入渗系数 分区面积/km2 岩溶 0.50 0.419 有水落水洞 0.65 0.297 落水洞 0.60 0.901 岩溶裂隙 0.25 0.233 山顶 0.2 0.373 河流 0.15 0.116 
下载: 导出CSV
-
[1] Goldscheider N, Meiman J, Pronk M, Smart C. Tracer tests in karst hydrogeology and speleology[J]. International Journal of Speleology, 2008, 37(1). [2] 李宗发. 贵州喀斯特地貌分区[J]. 贵州地质, 2011, 28(3):177-181, 234. doi: 10.3969/j.issn.1000-5943.2011.03.005LI Zongfa. Division of Karstlandform in Guizhou[J]. Guizhou Geology, 2011, 28(3): 177-181. doi: 10.3969/j.issn.1000-5943.2011.03.005 [3] 曹建华, 袁道先, 章程, 章程, 蒋忠诚. 受地质条件制约的中国西南岩溶生态系统[J]. 地球与环境, 2004, 32(1):1-8. doi: 10.3969/j.issn.1672-9250.2004.01.001CAO Jianhua, YUAN Daoxian, ZHANG Cheng, JIANG Zhongcheng. Karst ecosystem constrained by geological conditions in Southwest China[J]. Earth and Environment, 2004, 32(1): 1-8. doi: 10.3969/j.issn.1672-9250.2004.01.001 [4] 谭家华. MODFLOW-CFP软件在岩溶水系统数值模拟应用中的若干关键问题[J]. 中国岩溶, 2023, 42(4):636-647. doi: 10.11932/karst20230402TAN Jiahua. Several key issues in the application of MODFLOW-CFP software to the numerical simulation of karst water systems[J]. Carsologica Sinica, 2023, 42(4): 636-647. doi: 10.11932/karst20230402 [5] 陈崇希. 岩溶管道-裂隙-孔隙三重空隙介质地下水流模型及模拟方法研究[J]. 地球科学, 1995, 20(4):361-366.CHEN Chongxi. Groundwater flow model and simulation method in triple media of karstic tube-fissure-pore[J]. Earth Science, 1995, 20(4): 361-366. [6] 徐中平, 周训, 崔相飞, 拓明明, 王昕昀, 张颖. 岩溶区地下水数值模拟研究进展[J]. 中国岩溶, 2018, 37(4):475-483. doi: 10.11932/karst20180401XU Zhongping, ZHOU Xun, CUI Xiangfei, TA Mingming, WANG Xinyun, ZHANG Ying. Research advances of numerical simulation of groundwater in karst areas[J]. Carsologica Sinica, 2018, 37(4): 475-483. doi: 10.11932/karst20180401 [7] 董贵明, 束龙仓, 田娟, 季叶飞. 西南岩溶地下河系统水流运动数值模型[J]. 吉林大学学报(地球科学版), 2011, 41(4): 1136-1143.DONG Guiming, SHU Longcang, TIAN Juan, JI Yefei. Numerical Model of Groundwater Flow in Karst Underground River System, Southwestern China[J]. Journal of Jilin University. Earth Science Edition, 2011, 41(4): 1136-1143, 1156. [8] Hartmann A, Goldscheider N, Wagener T, Lange J, Weiler M. Karst water resources in a changing world: Review of hydrological modeling approaches[J]. Reviews of Geophysics, 2014, 52(3): 218-242. doi: 10.1002/2013RG000443 [9] Shoemaker W, Kuniansky E, Birk S, Bauer S, Swain E. Documentation of a conduit flow process (CFP) for MODFLOW-2005[J]. 2008. [10] 闵佳. “渗流—管流耦合模型”的物理模拟及数值模拟[D]. 北京:中国地质大学(北京), 2020.MIN Jia. Physical simulation and numerical simulation of "seepage-tube coupling model"[D]. Beijing: China University of Geosciences(Beijing), 2019. [11] Li C, Xing L, Dong Y, Peng Y, Xing X, Li C, Zhao Z. Numerical simulation and protection of the dynamic change of Jinan karst spring based on coupling of seepage and conduit flow[J]. Heliyon, 2022, 8(9): e10428. doi: 10.1016/j.heliyon.2022.e10428 [12] 赵良杰, 夏日元, 杨杨, 邵景力, 曹建文, 樊连杰. 基于CFP的岩溶管道流数值模拟研究:以桂林寨底地下河子系统为例[J]. 地球学报, 2018, 39(2):225-232. doi: 10.3975/cagsb.2017.112502ZHAO Liangjie, XIA Riyuan, YANG Yang, SHAO Jingli, CAO Jianwen, FAN Lianjie. Research on Numerical Simulation of Karst Conduit Media Based on CFP: A Case Study of Zhaidi Karst Underground River Subsystem of Guilin[J]. Acta Geoscientia Sinica, 2018, 39(2): 225-232. doi: 10.3975/cagsb.2017.112502 [13] 杨杨, 赵良杰, 潘晓东, 夏日元, 曹建文. 西南岩溶山区地下水资源评价方法对比研究:以寨底地下河流域为例[J]. 中国岩溶, 2022, 41(01):111-123. doi: 10.11932/karst20220106YANG Yang, ZHAO Liangjie, PAN Xiaodong, XIA Riyuan, CAO Jianwen. Comparative study on evaluation methods of groundwater resources in karst area of Southwest China: Taking Zhaidi underground river basin as an example[J]. Carsologica Sinica, 2022, 41(01): 111-123. doi: 10.11932/karst20220106 [14] 贺鹏, 贺颜, 张晓. 基于GMS隧道渗流场特征及涌水量数值模拟[J]. 陕西水利, 2023(2):28-29,35. doi: 10.3969/j.issn.1673-9000.2023.2.shanxsl202302009HE Peng, HE Yan, ZHANG Xiao. Numerical Simulation of Seepage Field Characteristics and Water Inflow of Tunnel Based on GMS[J]. Shaanxi Water Resources, 2023(2): 28-29,35. doi: 10.3969/j.issn.1673-9000.2023.2.shanxsl202302009 [15] 高国庆, 齐国庆, 陈仲达, 刘剑锋. 基于GMS的红崖山隧道渗流场分析与涌水量预测[J]. 水利与建筑工程学报, 2022, 20(4):142-148,211. doi: 10.3969/j.issn.1672-1144.2022.04.021GAO Guoqing, QI Guoqing, CHEN Zhongda, LIU Jianfeng. Analysis of Seepage Field and Prediction of Water Inflow in Hongyashan Tunnel Based on GMS[J]. Journal of Water Resources and Architectural Engineering, 2022, 20(4): 142-148,211. doi: 10.3969/j.issn.1672-1144.2022.04.021 [16] 康志强, 张勤军, 刘玲, 贝为昶, 袁金福, 梁爽, 黄春阳. 基于MODFLOW的贺江岩溶流域地下水资源评价[J]. 桂林理工大学学报, 2023: 1-9.KANG Zhiqiang, ZHANG Qinjun, LIU Ling, BEI Weichang, YUAN Jinfu, LIANG Shuang, HUANG Chunyang. Evaluation of groundwater resources based on MODFLOW in Hejiang Karst basin [J]. Journal of Guilin University of Technology, 2023: 1-9. [17] 肖喜. 重庆东泉隧道岩溶地下水动力特征及围岩防突厚度研究[D]. 成都:西南交通大学, 2024.XIAO Xi. Study on dynamic characteristics of karst groundwater and thickness of surrounding rock outburst prevention of Dongquan tunnel in Chongqing[D].Chengdu:Southwest Jiaotong University,2024. [18] 周焱钰, 陈喜, 张志才. 西南喀斯特小流域地下水数值模拟[J]. 工程勘察, 2011, 39(4):43-47,51.ZHOU Yanyu, CHEN Xi, ZHANG Zhicai. Numerical groundwater modeling in a small karst watershed of the Southwest China[J]. Geotechnical Investigation & Surveying, 2011, 39(4): 43-47,51. [19] 张子琦, 许模, 曹聪, 张强, 夏强. MODFLOW岩溶暗河及出口的概化与模拟效果[J]. 地质科技通报, 2023, 42(4): 250-258.ZHANG Ziqi , XU Mo, CAO Cong, ZHANG Qiang, XIA Qiang. Conceptualization and numerical simulation of a karst subterranean river and its outlets using MODFLOW[J]. Bulletin of Geological Science and Technology, 2023, 42(4): 250-258. [20] 罗利川, 梁杏, 李扬, 周宏, 罗明明. 基于GMS的岩溶山区三维地下水流模式识别[J]. 中国岩溶, 2018, 37(5):680-689. doi: 10.11932/karst20180505LUO Lichuan, LIANG Xing, LIANG Xing, ZHOU Hong, LUO Mingming. Identifying three-dimensional groundwater flow patterns[J]. Carsologica Sinica, 2018, 37(5): 680-689. doi: 10.11932/karst20180505 [21] 党志文, 邵景力, 崔亚莉, 李军, 宫志强, 赵良杰, 梁永升. 基于MODFLOW-CFP的贵州大井流域岩溶地下水数值模拟[J]. 中国岩溶, 2023, 42(2):266-276. doi: 10.11932/karst2023y002DANG Zhiwen, SHAO Jingli, CUI Yali, LI Jun, GONG Zhiqiang, ZHAO Liangjie, LIANG Yongsheng. Numerical simulation of karst groundwater in Dajing basin of Guizhou Province based on MODFLOW-CFP[J]. Carsologica Sinica, 2023, 42(2): 266-276. doi: 10.11932/karst2023y002 [22] 高恒. 贵州平寨水库库区岩溶地下水数值模拟及地下水库库容分析[D]. 北京:中国地质大学(北京), 2022.GAO Heng. Numerical Simulation of Karst Groundwater and Analysis of Groundwater Reservoir Capacity in Pingzhai Reservoir Area, Guizhou[D]. Beijing: China University of Geosciences(Beijing), 2022. [23] 杨杨, 赵良杰, 苏春田, 夏日元. 基于CFP的岩溶管道流溶质运移数值模拟研究[J]. 水文地质工程地质, 2019, 46(4):51-57.YANG Yang, ZHAO Liangjie, SU Chuntian, XIA Riyuan. A study of the solute transport model for karst conduits based on CFP[J]. Hydrogeology & Engineering Geology, 2019, 46(4): 51-57. [24] Gallegos J J. Modeling Groundwater Flow in Karst Aquifers: An Evaluation of MODFLOW-CFP at the Laboratory and Sub-Regional Scales[J]. 2011. [25] 马从文, 张志才, 陈喜, 程勤波, 彭韬, 张林. 基于机器学习的西南岩溶泉流量模拟研究[J]. 中国岩溶, 2024, 43(1):48-56. doi: 10.11932/karst2023y013MA Congwen, ZHANG Zhicai, CHEN Xi, CHENG Qinbo, PENG Tao, ZHANG Lin. Modelling karst spring flow in Southwest China based on machine learning[J]. Carsologica Sinica, 2024, 43(1): 48-56. doi: 10.11932/karst2023y013 [26] Chu X, Ding H, Zhang X. Simulation of solute transport behaviors in saturated karst aquifer system[J]. Scientific Reports, 2021, 11(1): 15614. doi: 10.1038/s41598-021-94950-7 [27] 赵小二, 常勇, 吴吉春. 岩溶地下河污染物运移模型对比研究[J]. 环境科学学报, 2020, 40(4):1250-1259.ZHAO Xiaoer, CHANG Yong, WU Jichun. A comparative study on two contaminant transport models used in karst underground rivers[J]. Acta Scientiae Circumstantiae, 2020, 40(4): 1250-1259. [28] 赵小二, 王正一, 武桂芝, 李琪. 弯曲岩溶管道溶质运移的尺度效应研究[J]. 水文地质工程地质, 2023, 50(2):44-53.ZHAO Xiaoer, Wang Zhengyi, WU Guizhi, LI Qi. Research on the scale effects of solute transport in a bended karst conduit[J]. Hydrogeology & Engineering Geology, 2023, 50(2): 44-53. [29] 郑小康, 杨志兵. 岩溶含水层饱和-非饱和流动与污染物运移数值模拟[J]. 地质科技通报, 2022, 41(5):357-366.ZHENG Xiaokang, YANG Zhibing. Numerical simulation of saturated-unsaturated groundwater flow and contaminant transport in a karst aquifer[J]. Bulletin of Geological Science and Technology, 2022, 41(5): 357-366. [30] Davis J H, Katz B G, Griffin D W. Nitrate-N movement in groundwater from the land application of treated municipal wastewater and other sources in the Wakulla Springs springshed, Leon and Wakulla Counties, Florida, 1966-2018: 2010-5099[R] Scientific Investigations Report. U. S. Geological Survey, 2010. [31] Peng Shouzhang, Ding Yongxia, Liu Wenzhao, Li Zhi. 1 km monthly temperature and precipitation dataset for China from 1901 to 2017. Earth System Science Data, 2019, 11, 1931−1946. https://doi.org/10.5194/essd-11-1931-2019. [32] Shah N, Nachabe M, Ross M. Extinction Depth and Evapotranspiration from Ground Water under Selected Land Covers[J]. Groundwater, 2007, 45(3): 329-338. doi: 10.1111/j.1745-6584.2007.00302.x [33] 张人权. 水文地质学基础[M]. 北京:地质出版社, 2018.ZHANG Renquan. Fundamentals of Hydrogeology (7th Edition) [M]. Beijing: Geology Press, Beijing, 2018. [34] 任婉立. 非均质孔隙介质中溶质运移模拟的不确定性分析[D]. 长春:吉林大学, 2023.REN Wanli. Uncertainty analysis of solute transport simulation in heterogeneous porous media[D]. Changchun: Jilin University, 2023. -
点击查看大图
图(17) / 表(2)
计量
- 文章访问数: 14
- HTML浏览量: 3
- PDF下载量: 8
- 被引次数: 0
